Joséphin Bastière

🇫🇷 🇬🇧 🇪🇸

0 € 0

Shopping Cart

0
No products in the cart.
Menu

🇫🇷 🇬🇧 🇪🇸

0 € 0

Shopping Cart

0
No products in the cart.
Menu

The HyperBrut art
- Map of maps -

In my various adventures with HyperBrut art, in the middle of 2016, while trying to sort through my drawings, paintings, writings, I discovered the Map of Maps :

I gave it the name Map of Maps because the purpose of this thing is to map everything that is mappable, or in other words, to represent everything that is representable, to design everything that is conceivable. At first, it looked like this and was used to sort my drawings :
Then I gradually understood that this map is not flat but spherical, a sphere of infinite dimension :
And that we can only see it partially.
 
This map is composed of an inconceivable background, in which there is what is inconceivable, unimaginable, unrepresentable, then in the center is the field of the conceivable, the imaginable, the representable. The first thing that appears in the center of this map is a dot:
The next layer consists of a line ( series of points aligned in one direction ), then two lines ( two series of points each aligned in two distinct directions ), and three lines ( three series of points each aligned in three distinct directions ), which will correspond respectively to the worlds of line, plane and volume :
And what will appear in the next layer, all of this will depend on these worlds of line, plane and volume. Like for example rock art, the first traces of representations made by humans, this art will depend on lines in plans on volumes :

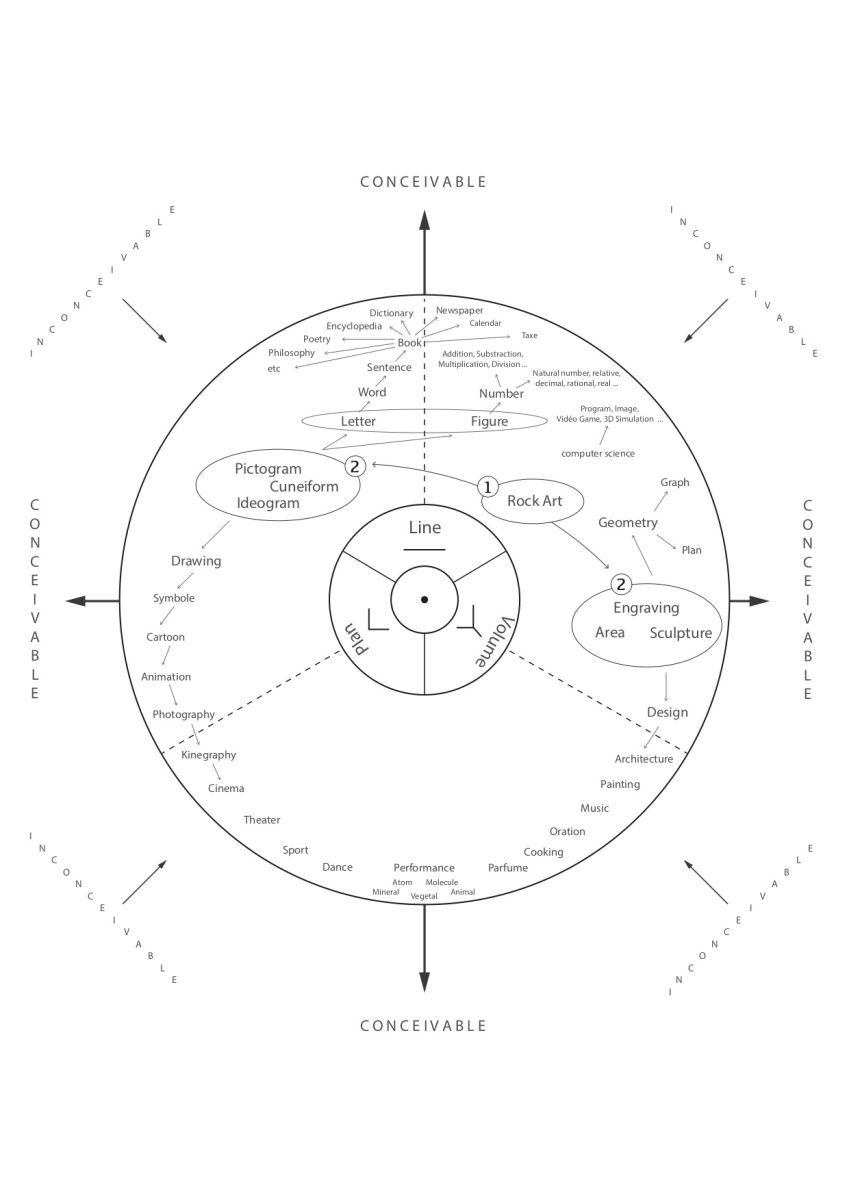
Another example would be W.A. Mozart’s symphony number 40. Let’s admit that the best representation of this symphony is the score itself. So in this case, this partition is in a mix between pictogram, letter, number, carved on a volume which would be the sheets of paper constituting the partition. Now let’s say that the best representation of this symphony is when so-and-so orchestra played at so-and-so. So in this case, this performance is in a mixture of performance, having taken place in a building structured in such and such a way to accommodate the orchestra which will sculpt the air. Let us now admit that the best representation of this symphony is the very idea that Mozart had of this work. So in this case, this representation is in the performance that is the living sculpture of Mozart himself.

In each case, it’s like zooming in on the Map of Maps. In the case of the score it seems almost flat, in two dimensions, then in the case of the orchestra or Mozart it involves more volume. But the line, the plan, and the volume are all three present in the representations of symphony number 40. And finally, isn’t it all of these representations that allow us to have a more precise idea of this symphony ?

I can take another example. What would be the best representation of a chair ? Could it be the drawing of some chair ? Or the design of your favorite chair ? Or would this be the set of all the chair designs that could have been made ? Or would it not be the volume that is the chair itself ? Or maybe the best representation would be the one we can each make of a chair.

One example I experienced when I was discovering this map was the example of my school. I wondered what was the best representation of my school when I was a kid. I found that the set of building plans on each floor, could give a good representation of the school. I realized that another good representation of the school was the sculpture that was the school. Other good representations of the school would have been photographs over time, or the architect’s drawing before the school was built, or the representation of the person who commissioned the building. Or another representation of the school could have been a novel that told the story of the building. And so on.

Thus all the representations of world maps or any other representation that you may have come across in your life are found in this Map of maps. And this map of the world or other representation, would be a precise section in the Map of maps between volume, plan, and line.

Small remark to say that it happens regularly in our life, to see inconceivable things appear in the field of the conceivable, hence the direction of the axes.

Now, to better understand what this point in the center of this Map of Maps looks like, I offer two examples. In the image below, I drew a black dot for you on a white background :

That is to say, I really wanted to draw a black dot on a white background, but since the dot has no thickness, I wanted to do it so well that in the end I didn’t put my pen on the sheet.

Now, in the image below, I’ve drawn you a white dot on a black background :

That is to say, I really wanted to draw a white dot on a black background, but as soon as I wanted to draw it, the support that I was using to draw it, this support was already encompassed by the white dot.
 
 These two examples taken together give an overview of what this point in the center of the Map of maps is, and allow to understand that this point includes what is above the volume, and below the plane, the line, see even from the point.
 
 And of course, it is possible to add to these two examples their soul mate, that is to say white dot on black background, and black dot on white background :

There you go, here you are with a description of the Map of Maps. I have described this to you in spite of the other quirks that I have encountered in HyperBrut art and HyperBrutalism, because this Map of Maps allows you to have an overview of all Brut-Gestures and because it it is with her and her point in the center that I was able to discover Univers 0.